The first step is to set up a topological band theory of vibrational modes by defining the Fourier-transformed rigidity matrix \(R(\mathbf{k})\) whose determinant is complex: \(\det R(\mathbf{k}) \equiv |\det R(\mathbf{k})|e^{i\phi(\mathbf{k})}\). Consider now for simplicity a two dimensional lattice whose phonons are gapped, away from \(\mathbf{k}=\mathbf{0}\), like the twisted Kagome lattice shown at the end of the introductory video.
Two winding numbers, \(n_i=\{n_1,n_2\}\), of \(\phi(\mathbf{k})\) can be evaluated using
\[ n_i=- \frac{1}{2\pi}\oint_{C_i}d\mathbf{k}\cdot\nabla_\mathbf{k} \phi(\mathbf{k}) \]
along the two cycles \(\{C_1,C_2\}\) of the Brillouin zone corresponding to the reciprocal lattice directions orthogonal to the basis vectors \(\{\mathbf{a}_1,\mathbf{a}_2\}\) of the lattice. The winding numbers are invariants of the gapped lattice; smooth deformations do not change \(n_i\) unless the lattice becomes untwisted and the phonon gap closes along a line in the Brillouin zone. We can now define the topological polarization
\[ \mathbf{P}_T = \sum_i n_i \mathbf{a}_i. \]
In the figure below, the topological polarization of the deformed Kagome lattice is \(\mathbf{P}_T = \mathbf{a}_1\) in terms of the primitive vectors \(\mathbf{a}_i\).
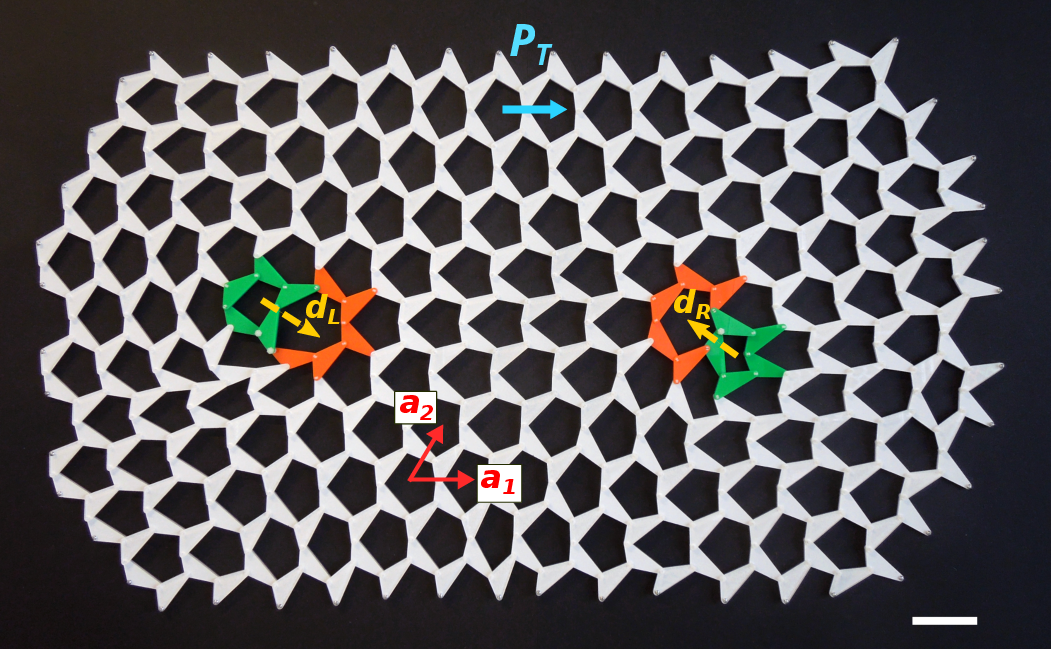
Kane and Lubensky proved that the number of topological zero modes (i.e. modes that arise without adding or removing bonds anywhere in the structure) minus the number of topological states of self stress, \(\nu_\text{T}\), in any lattice subsystem bounded by \(\cal C\) is given by
\[ \nu_\text{T} = \oint_{\cal C} \frac{d^{d-1}S}{V_\text{cell}} \mathbf{P}_T \cdot\hat{n}, \]
where \(\hat{n}\) is the inward-pointing normal to the boundary, and \(V_\text{cell}\) is the \(d\)-dimensional volume of the unit cell.
Notice that this result applies to a patch of the material, unlike the original Maxwell count which is a global statement. It can be understood intuitively with the aid of the electrostatic analogy to polarized media. Just as Gauss’s law yields the net charge enclosed in a region from the flux of the electric polarization through its boundary, the net value of \(\nu_\text{T}\) in an arbitrary portion of an isostatic lattice is given by the flux of the topological polarization through its boundary.